Page 2 - LoRe_Musumeci_alii_2014

C. Lo Re et al. / Procedia Engineering 70 ( 2014 ) 1046 – 1054 1047

For example, Baldock and Holmes (1999) found in numerical simulations that incident band swash saturation
was related to bore-driven swash, which also scales with wave period and beach slope. Moreover, Bellotti and
Brocchini (2005) present simulations of the shoreline displacement based on bore run-up theory which give
excellent agreement with experimental data for regular waves, wave groups and random waves.
Moreover, a recent numerical investigation concerning the simulation of swash zone fluid accelerations,
concluded that given the poor correlation between local acceleration and pressure gradient, it is not likely that local
acceleration can be used as a surrogate for pressure gradient (Puleo et al., 2007).
Notwithstanding the advances of the research, numerical models are often calibrated by using datasets produced
during laboratory experiments (Xuan et al. 2013, Ramirez et al. 2013, Li et al. 2012 ). Although the analysis of
laboratory results can provide some insights on what happens in Nature, the processes occurring in the field may be
quite different from lab experiments due to many causes, e.g. the use of idealized wave conditions, such as regular
waves, or turbulence-related scale effects which can be hardly eliminated in the lab.
To become a practical tool of engineering interest, the performance of a numerical model should be compared to
field data conditions, where the effects of phenomena such as the actual irregularity of wave trains, presence of
turbulence, infiltration and ex-filtration processes are included.
To contribute to fill such a gap, in the present work a comparison between the numerical results obtained by the
Lagrangian shoreline model coupled with a Boussinesq type model proposed by Lo Re et al. (2012) and the results
of a field campaign on wave run up on a mild slope sandy beach is discussed.
The paper is organized as follows: the next Section provides a description of the numerical model; then Section
3 describes the field campaign; Section 4 presents the procedures used to input the data in the numerical models,
based on field data, and to analyze the results; Section 5 illustrates and discuss the obtained results; finally Section
6 draws the conclusions of the work.
2. Methods

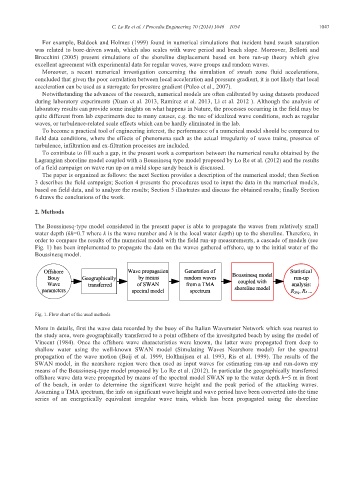
The Boussinesq-type model considered in the present paper is able to propagate the waves from relatively small
water depth (kh=0.7 where k is the wave number and h is the local water depth) up to the shoreline. Therefore, in
order to compare the results of the numerical model with the field run-up measurements, a cascade of models (see
Fig. 1) has been implemented to propagate the data on the waves gathered offshore, up to the initial water of the
Boussinesq model.

Fig. 1. Flow chart of the used methods

More in details, first the wave data recorded by the buoy of the Italian Wavemeter Network which was nearest to
the study area, were geographically transferred to a point offshore of the invesitgated beach by using the model of
Vincent (1984). Once the offshore wave characteristics were known, the latter were propagated from deep to
shallow water using the well-known SWAN model (Simulating Waves Nearshore model) for the spectral
propagation of the wave motion (Boij et al. 1999, Holthuijsen et al. 1993, Ris et al. 1999). The results of the
SWAN model, in the nearshore region were then used as input waves for estimating run-up and run-down my
means of the Boussinesq-type model proposed by Lo Re et al. (2012). In particular the geographically transferred
offshore wave data were propagated by means of the spectral model SWAN up to the water depth h=5 m in front
of the beach, in order to determine the significant wave height and the peak period of the attacking waves.
Assuming a TMA spectrum, the info on significant wave height and wave period have been converted into the time
series of an energetically equivalent irregular wave train, which has been propagated using the shoreline