Page 3 - LoRe_Musumeci_alii_2014

1048 C. Lo Re et al. / Procedia Engineering 70 ( 2014 ) 1046 – 1054

Lagrangian numerical model of Lo Re et al. (2012) in a numerical flume having the same beach slope of the
measured transect (Fig.s 2).

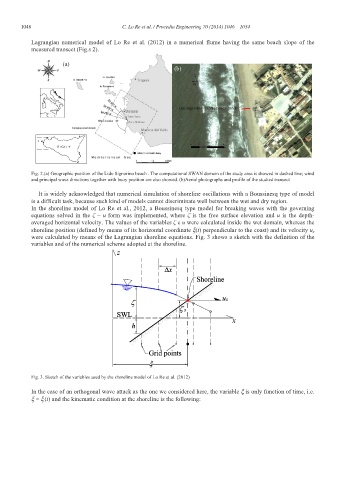
(a)
(b)

Fig. 2.(a) Geographic position of the Lido Signorino beach . The computational SWAN domain of the study area is showed in dashed line; wind
and principal wave directions together with buoy position are also showed. (b)Aerial photographs and profile of the studied transect

It is widely acknowledged that numerical simulation of shoreline oscillations with a Boussinesq type of model
is a difficult task, because such kind of models cannot discriminate well between the wet and dry region.
In the shoreline model of Lo Re et al., 2012, a Boussinesq type model for breaking waves with the governing
equations solved in the ζ – u form was implemented, where ζ is the free surface elevation and u is the depth-
averaged horizontal velocity. The values of the variables ζ e u were calculated inside the wet domain, whereas the
shoreline position (defined by means of its horizontal coordinate ξ(t) perpendicular to the coast) and its velocity u s
were calculated by means of the Lagrangian shoreline equations. Fig. 3 shows a sketch with the definition of the
variables and of the numerical scheme adopted at the shoreline.

Fig. 3. Sketch of the variables used by the shoreline model of Lo Re et al. (2012)

In the case of an orthogonal wave attack as the one we considered here, the variable ξ is only function of time, i.e.
ξ = ξ (t) and the kinematic condition at the shoreline is the following: